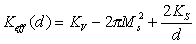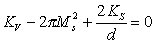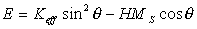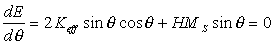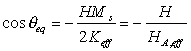2. Short tutorial on magnetism of nanostructures
Now technologies allow us to create artificial materials and
nanostructures which are absent in the Nature. Such nanostructures could
be built on the atomic level by a precise deposition of small group
atoms (atomic layers) and even isolated atoms on a specially prepared
substrate. During the deposition one can combine atoms of different
chemical elements, for example, to produce sandwiches from magnetic and
non-magnetic atomic layers or sandwiches of a few magnetic atomic layers
covered by layers of non-magnetic metals (see Fig.2.1).

|
| Fig. 2.1. Quasi-2D nano-sandwich constructed from the atomic layers of
different chemical elements (the different elements marked in different
colors). |
Magnetic properties of such nanoobjects are quite unusual and puzzled.
Moreover, such magnetic nanostructure, e.g. ultrathin magnetic films
have a huge application potential, e.g. in magnetic memory storage and
recording devices.
|
|
Fig. 2.2. Magnetic cobalt nanowedge (schematically). The arrows
show the orientation of magnetization vector. On the left (thinner
part), the magnetization state is perpendicular one, while on the right
(thicker part), in-plane. The contributions from the "top" and
"bottom" surfaces to magnetic anisotropy are shown.
|
Magnetic perpendicular anisotropy of ultrathin ferromagnetic films is
one of fundamental interests of magnetism of low dimensional systems
(with dimensions between 2D and 3D). Magnetization states, depending on
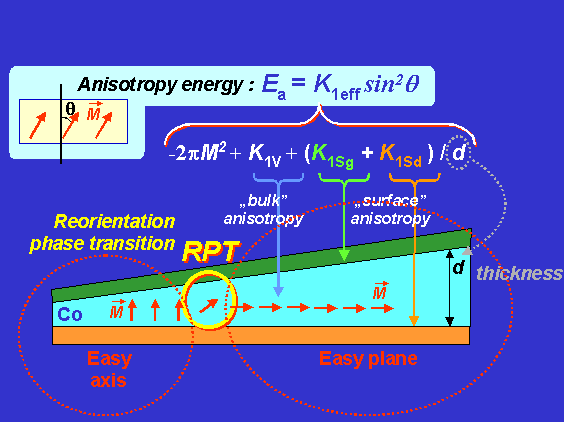
film thickness, are illustrated in Fig.2.2. For ultrathin film the
anisotropy energy EA is described in the following form
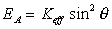 .
.
There are different sources for the anisotropy rising: crystalline (or
"bulk" anisotropy), the surface anisotropy due to the broken symmetry;
shape anisotropy and etc. These contributions in different ways depend
on the film thickness. It is commonly assumed to describe the thickness
dependence of the effective anisotropy energy of ultrahin films as
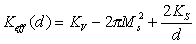
|
(2.1) |
where KV is the bulk "volume" anisotropy constant, Ks is the surface anisotropy constant. The second term in Eq.2.1 describes the
demagnetization energy which is -2 Pi MS2, for film in the
monodomain state. It is possible to realize vanishing of the constant
Keff (/e.g. changing the film thickness)
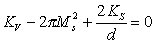
|
(2.2) |
So, at d=d1 (as Keff (d1 )=0). For cobalt films, depending on
the grown condition, substrates and coverage, d1 typically varies
from 1.5 to 1.8 nm.
Domain structures and magnetization processes for Co in an out-off plane
state (d < d1 ) differ from those which observed in micron-sized films.
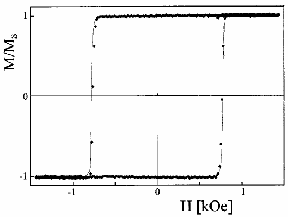
Such films may exhibit a square type of hysteresis loop shown in Fig.
3.2. Domain structures could be formally described using the models from
the previous chapter, see Eqs.1.14 and Eq.A2 and Eq.A3. It gives the
domain period in the range from ~100 km to hundreds of nanometers as the
cobalt thickness increases from d=0.8 nm to d1 ~= 1.79 nm [1].
However, for thinner films the domain structure geometry is mainly
governed by coercivity rather than magnetostatic forces. Fig. 2.3 shows
the domain structure registered without magnetic field in the cobalt
film with d=0.8 nm [2] (d < d1)). One can see the domain size is
much smaller than that predicted by the above theories. Such magnetic
domain states are out-of-equilibrium but they are long-time living what
could be very useful for applications. It is necessary to use quite
different physical basis to describe domain geometry and magnetization
processes - taking into account a spatial coercivity distribution one
can numerically simulate domain structures in ultrahin films. The result
of such simulations is shown in Fig.2.4 [2].
For d>d1 the magnetization vector lies into the film plane
and the film becomes in-plane magnetized. There are no magnetic domains
caused by magnetostatics. It is easy to describe magnetization process
for d>d1. Let us consider a simplified case allowing the
magnetization vector to coherently rotate in a homogeneously magnetized
film. In this case the total film energy is given by
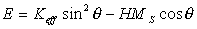
|
(2.3) |
where θ is the angle between the normal to the film surface and the
magnetization vector, H is the magnetic field applied along the film
normal. In equilibrium the inclination of magnetization from the film
normal corresponds to the minimum of the energy (Eq.2.3). So,
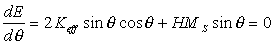
|
(2.4) |
By solution of Eq.2.4 we find the equilibrium magnetization
orientation, θe as
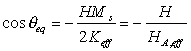
|
(2.5) |
where the normalized magnetization component perpendicular to the film
plane linearly depend on magnetic field normalized by the anisotropy
field, HA,eff = 2 Keff / Ms .

|
|
|
Fig. 2.3. Domain structure of ultrathin cobalt with d=0.8 nm and the
magnetic hysteresis loop obtained with using the Kerr effect [2].
|

|
|
Fig. 2.4. Simulated domain structure of ultrathin cobalt with d=0.8 nm
[2].
|
References
- M. Kisielewski, A. Maziewski, T. Polyakova, and V. Zablotskii.
Wide-scale evolution of magnetization distribution in ultrathin films.
/Phys. Rev. B/ 69, 184419 (2004).
- J. Ferré, V. Grolier, P. Meyer, S. Lemerle, A. Maziewski, E.
Stefanowicz, S. V. Tarasenko, V. V. Tarasenko, M. Kisielewski and D.
Renard. Magnetization reversal processes in an ultrathin Co/Au film
/Phys. Rev. B,* */55, 22, 15 092 (1997).
';
print '
Back to previous page ';
?>



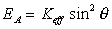 .
.